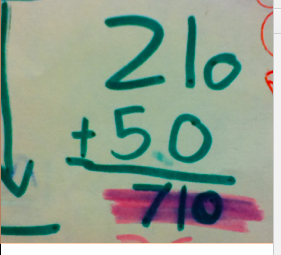We began the day by discussing the reading on linear relationships. Beth emphasized the idea that the proportional relationships we had been analyzing on Tuesday are really a special case of linear relationships: All proportional relationships are linear, but not all linear relationships are proportional. The proportional relationships are linear relationships with a zero intercept – in other words, the graph of the relationship passes through the origin.
Next, we dived into the “Burning the Candle” problem. Everyone was able to find numerical answers quickly, but it was harder to come up with the formula that describes this situation.
Resources discussed in the pedagogy discussion:
Doc Jul 20, 2017, 11_42-1xkyp0o
At the end of lunch, we took a group photo! Here it is:
In the afternoon, we examined a large set of relationships and decided whether or not they were linear. If they were linear, we decided whether or not they were proportional. We summarized by describing how we could recognize relationships:
For a Proportional relationship:
- The graph must be a line and must pass through (0,0).
- There must be a constant rate of change.
- The two quantities must have a constant ratio
For a linear relationship:
- The graph must be a line, but it does not need to pass through (0,0)
- There must be a constant rate of change.
- The two quantities do not necessarily have a constant ratio
For a relationship that is not linear:
- The graph doesn’t make a line
- There can be an exponent other than 1 or 0 (you can think about this one if you’re comfortable with the idea of exponents)
Notes from the afternoon pedagogy discussion:
A rich task…
- More than one way to approach the problem
- Opportunity for revision
- Not focused exclusively on procedures
- Connects to real life
- Independent/cooperative learning
- Analyze others’ solutions
- Encourages math discourse
- Productive struggle
- Builds on prior knowledge
- Requires adequate time
- Engaging/Raises curiosity
- Address misconceptions
- Students creating their own problem.
Resources for Rich Tasks
-
- NCTM publications: Teaching Children’s Mathematics Journal
- NCTM Illumination: http://www.nctm.org/
- Illustrative Mathematics Project: https://www.illustrativemathematics.org/
- Mathematics Assessment Project
- Dan Meyer’s blog. Eg: Nana’s Chocolate Milk: http://threeacts.mrmeyer.com/nana/sequel/act1.mov
- Yummy Math: https://www.yummymath.com/
- NextLesson : https://www.nextlesson.org/
- LearnZillion: https://learnzillion.com/p/