I love the Research Notes of the AAS.  They are a place for very short, unrefereed articles through AAS Journals, edited (but not copyedited!) by Chris Lintott. They are a great place for the scraps of research—those little results you generate that don’t really fit into a big paper—to get formally published and read.

You might think that without peer review and with such a low bar for relevance, such a journal would have a very high acceptance rate, but actually I’ve read it’s the most selective of the entire AAS family of journals, including ApJL!  The things it publishes are genuinely useful, and shows that there’s a need for publishing models for good ideas that are too small to be worth the full machinery of traditional publishing.  The curation by Chris also ensures that the ideas really are interesting and worthy of publication.

A while back I wrote a Research Note on how to prove the Earth moves with just a telescope and a camera. Nothing that would leave to novel results, but it has inspired some amateurs to try it out!

For my latest note, I’ve got another trick you can do with nothing but a telescope and a camera, although in this case they’ll cost billions of dollars and do something useful and novel!

Whenever I hang out with Eric Mamajek we end up talking science and coming up with cool ideas. This often ends with one of us starting an Overleaf document for a quick paper that never ends up getting written.  But the idea we had on my last trip was good enough that I was determined to see it through!

The idea goes back to Eddington’s eclipse experiment, wherein he showed that a gravitational field deflects starlight at the level predicted by General Relativity (which is twice the level one might deduce from Newtonian gravity).

To do this, he imaged the sky during a total solar eclipse, when he could make out stars near the sun.  Comparing their positions to where they were measured during the night at other times of year, he showed they were significantly out of place, meaning the Sun had bent their rays. Specifically, he found that they were farther from the Sun by about an arcsecond (in essence, the Sun’s focusing effects allows us to see slightly behind it, and so everything around it appears slightly “pushed away” from its center.)

This led to a great set of headlines in the New York Times I like to show in class:

This is actually an example of what today we confusingly call microlensing. This actually captures a broad range of lensing effects, as Scott Gaudi explained to me here (click to read the whole thread).

the magnification pattern from individual stars. Galactic microlensing, where the images are separated by milliarcseconds, is just an abuse of the original term, and has sense come to mean cases where the images are unresolved, as @macyjhuston says.

— Scott Gaudi (@bsgaudi) September 20, 2023

Microlensing is most obvious when a source star passes almost directly behind a lens star—specifically within its Einstein radius, which is typically of order a few milliarcseconds. This level of alignment is very rare, but if you look at a dense field stars, like towards the Galactic Bulge, then there are so many potential lenses and sources that alignments happen frequently enough that you can detect them with wide-angle cameras.

In close alignments like this, the image of the background source star gets distorted, magnified, and multiply imaged, resulting in it getting greatly magnified in brightness, as shown in this classic animation by Scott.  Here, the orange circle is the background source star passing behind the foreground lens, shown by the orange asterisk. The green circle is the Einstein ring of the foreground lens.  As the background star moves within the Einstein ring, we see it as two images, shown in blue.

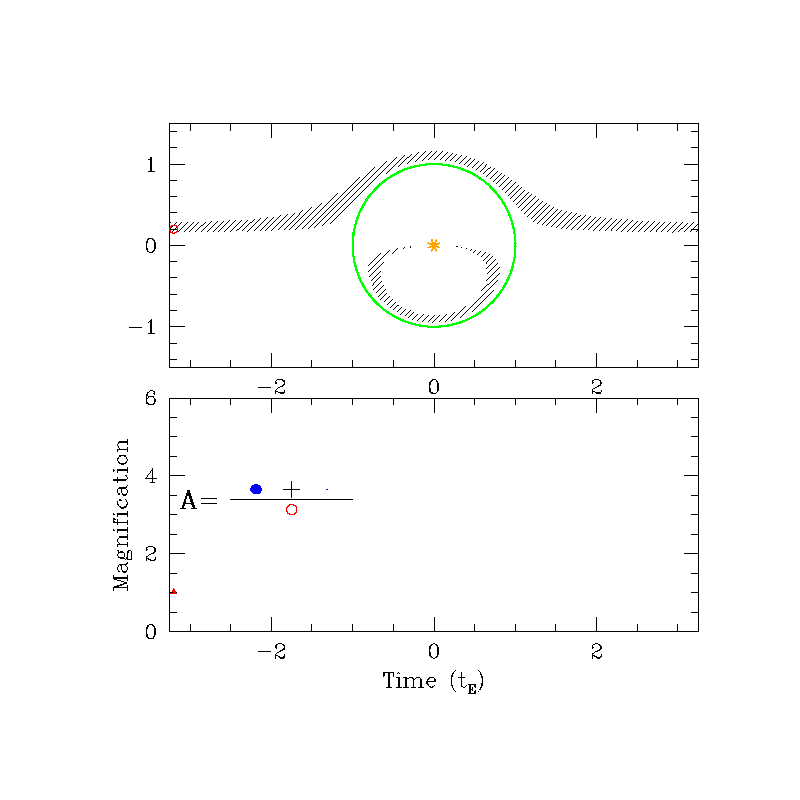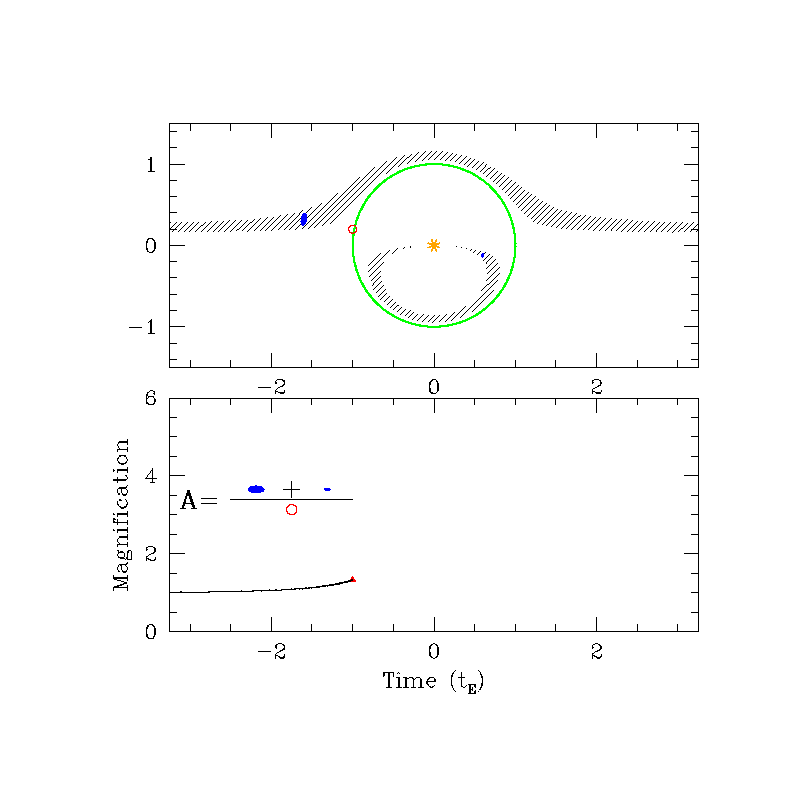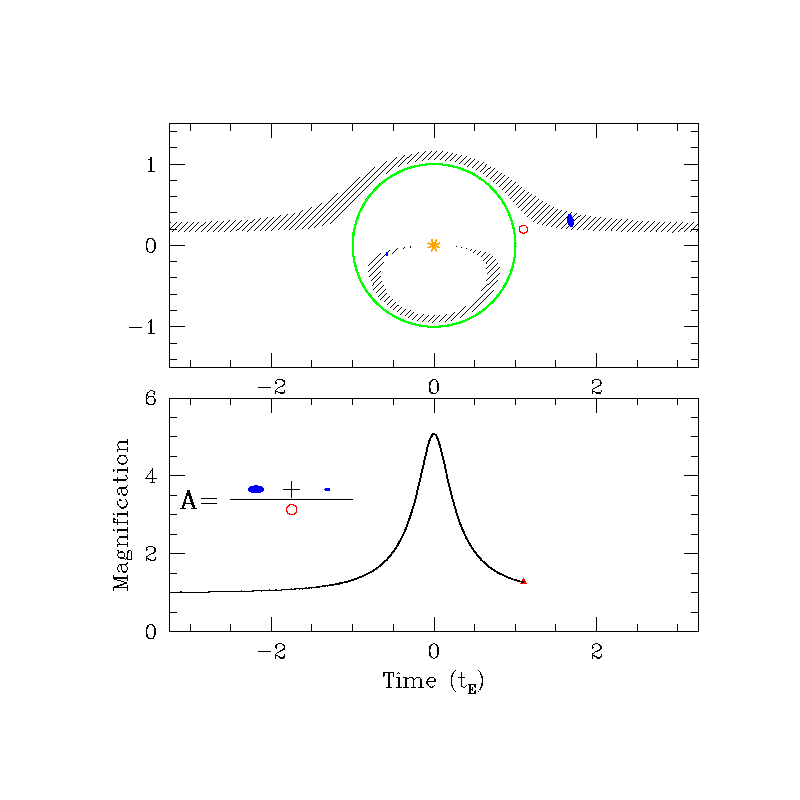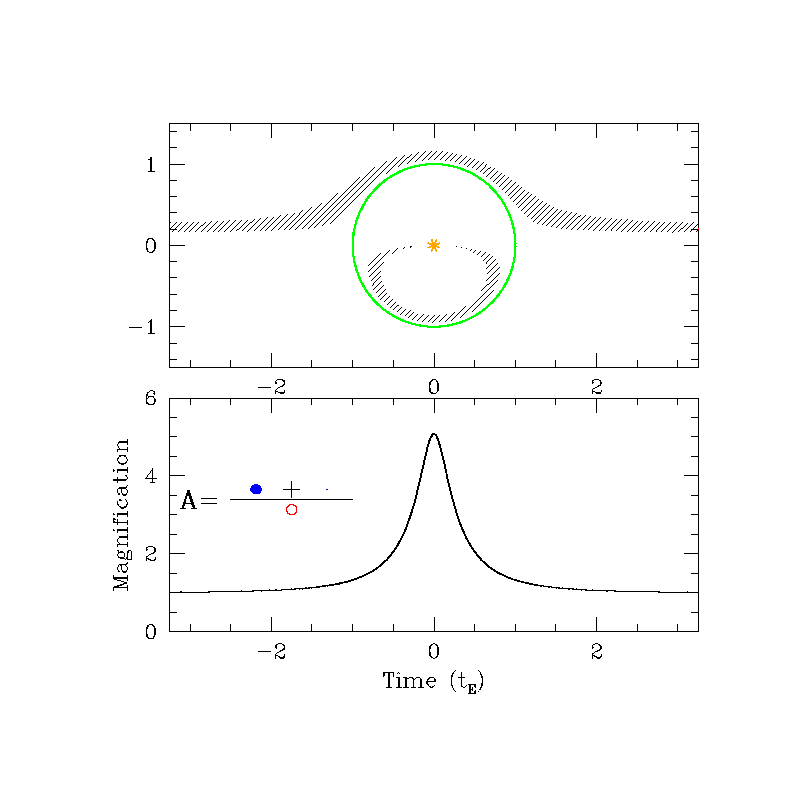
We typically do not resolve the detail seen in the top panel, we only see the total brightness of the system.  The brightness of just the background source is plotted in the bottom panel.

But in the rare cases where we can resolve the action, note what we can see: the background star is displaced away from the lens when it gets close, just like in Eddington’s experiment. This effect is very small, just milliarcseconds, and has only been measured a few times. This is called astrometric microlensing.

Rosie Di Sefano has a nice paper on what she dubs “mesolensing”: a case where instead of a rare occurence rate of lensing among many foreground objects, like in traditional microlensing surveys, you have a high rate of lensing for a single foreground object.  This occurs for very nearby objects moving against a background of high source density, like the Galactic Bulge.

The reason is that the Einstein ring radius of nearby objects is very large—for a nearby star it is of order 30 mas, or 0.”03.  Now, there is a very low chance of a background star happening to land so close to a foreground star, but foreground stars tend to move at several arcseconds per year across the sky, so the total solid angle (“area”) covered by the Einstein ring is actually a few tenths of a square arcsecond per year, which is starting to get interesting.

Things are even more interesting if you don’t require a “direct hit”, but consider background stars that get within just 1″ or so of the lens: even though it’s 30 Einstein radii away, the astrometric microlensing effect is still of order 1 mas, which is actually detectable!

Now, most of these background objects are very faint, so this isn’t really something you can exploit. Twice, people have used the alignment of a very faint white dwarf and some background stars to see this happen, and also once with the faint M dwarf Proxima. But most main sequence stars are so much brighter than the background stars, that their light will completely swap them.

But detecting very faint objects within a couple of arcseconds of bright stars is exactly the problem coronagraphy seeks to solve with the upcoming Habitable Worlds Observatory!  This proposed future flagship mission will block out the light of nearby stars and try to image the reflected light of Earth-like planets orbiting them.  And while it’s at it, it will see the faint stars behind the nearby one at distances of a few to dozens of Einstein radii.

So, for target stars in the direction of the Galactic Bulge, HWO will detect astrometric microlensing! And it will do this “for free”: it will be looking for the planets orbiting the star, anyway!

So, who cares? Is this just a novelty? Actually, it will be very useful: measuring the astrometric microlensing will directly yield the mass of the host star. This is great, because we have almost no way of doing this otherwise: we need to rely on models of stellar evolution, which are great but still require conversion to observables, which comes with systematic uncertainties of order a few %.  Directly measuring stellar masses will allow us to avoid those systematics, and better understand each star’s history—and that of its planetary systems.

Now, if we find planets with orbital periods of a few years or less, we can also measure the host star masses using Kepler’s Third Law, but this is an independent way to do this, and it also works on stars without planets. In principle, you could even go pointing HWO at all of the stellar mass objects towards the bulge to do this measurement, making it a pure stellar astrophysics engine (precise stellar masses don’t sell flagship missions like exoplanets do, though).

The final piece of this calculation was we needed to know what the background source density of HWO target stars were. As luck would have it, my recent advisee Dr. Macy Huston had just graduated, and the final chapter of their thesis is on a piece of Galactic stellar modeling software that does exactly this calculation for microlensing! It’s called SynthPop and you’ll hear about it soon, but in the meantime they were able to calculate how many background sources we expect from an example HWO architecture around likely HWO targets.

Macy finds that the best case of 58 Oph will likely have over 15 stars in the coronagraphic dark hole that will show astrometric microlensing, giving us a ~5% mass measurement of the star every visit. These numbers are very rough by the way—the precision could easily be better than this.

Anyway, this RNAAS was a lot of fun to write, and you can read all of the details in it here.

The bottom line is that HWO will be able to measure the masses of all sorts of stars towards the Galactic Bulge directly, with no model dependancies!

Enjoy!