Particle Dynamics & Fractal Coagulation Processes
Particles are extremely important in the natural environment and in engineered systems. Particles contribute to water turbidity and many engineered systems are designed to treat water through particle removal. Particles can enhance the transport of relatively insoluble chemicals sorbed on their surfaces, and through coagulation can facilitate carbon export to sediments.
My research over the past 20+ years has examined particle transport dynamics, including the transport of chemicals to particles, and the formation of larger, fractal particles through aggregation processes, and how these particles and processes can be characterized.
Large aggregates that form in the ocean, called marine snow, can rapidly form and sink, resulting potentially in the export of carbon to deep ocean sediments. The formation of these aggregates can thus be important in global ocean carbon balances and can affect the rate of carbon loss to deep sediments, thus potentially sequestering this carbon. Working we researchers at the University of California, Santa Barbara, we discovered a new type of particle that can form in the ocean (transparent exopolymer particles; TEP) that are responsible for the rapid formation of marine snow. More information: See the paper by Alldrege et al (1993).
Particles produced through aggregation are highly amorphous, non-spherical and fractal, and have three-dimensional fractal dimensions that span a range of ~1.1 to the maximum of 3. Previous methods for calculating fractal dimensions of inorganic aggregates have been based on the analysis of a single particle, but most systems of interest consist of a spectrum of particle sizes. In order to analyze average properties of aggregates in real coagulating suspensions with broad size distributions, we developed new spectral techniques (Jiang&Logan, 1996) to estimate fractal dimensions. Using these techniques, we demonstrated that there was no universality of the fractal dimension for aggregates formed from fluid shear and differential sedimentation processes. Fractal dimensions of microspheres formed in three different mixing environments (1995-Logan&Kilps), for example, were: 1.9, in a paddle mixer; 1.59 in a rolling cylinder; and 1.43 to 1.74 in a laminar shear device (depending on shear rate and salt concentration).
The fractal nature of these particles has been found to have important implications for calculations of particle properties such as their settling velocities and collision efficiencies. Fractal aggregates of inorganic microspheres, for example, settle an average of eight times faster than spheres of identical size and mass. Collision frequencies between large bacterial aggregates (100 um) and small microspheres (~ 1 um) were five orders-of-magnitude higher (Li&Logan, 1997) than predicted using sphere-based (curvilinear) coagulation models. Similarly high but slightly larger collision frequencies were obtained for aggregates made from microspheres. These results demonstrate that fractal aggregates of particles can collide much more frequently than expected based on spherical-particle coagulation models. They suggest that coagulation rates in natural and engineered systems are much more rapid than predicted by coagulation models based on impermeable spheres.
Use the links provided through this web page to see examples of fractals and to see a list of publications associated with this topic.
Macromolecules & Molecular Size Distributions
The study of the microbial breakdown of macromolecules is an important area of research for natural and engineered systems. The largest pool of organic carbon on the planet is the dissolved organic matter (DOM) in the ocean. Understanding the fate of this material can help us to understand global carbon cycling and the dynamics of natural systems. In engineered systems, such as water and wastewater treatment systems, the efficiency of removal of DOM in both abiotic and microbially-based systems is a function of the molecule size.
When is a organic matter a “molecule” or a “particle”? There is no one accepted size classification of DOM. For example, marine chemists define colloids as DOM greater than 1000 Daltons (1 kDa), and particles as material larger than 0.2 um. Water treatment engineers characterize colloids as particles less than 0.2 um but larger than 100 kDa, defining macromolecules as any DOM that cannot be classified (typically with a molecular weight of > 1 kDa).
Bacteria must degrade molecules larger than approximately 1 kDa before they can be taken into the cell and oxidized for energy. The breakdown of macromolecules, defined here as all material larger than 1 kDa, is a relatively unexamined area of research. It is difficult to conduct such research as the changes in size distributions of these molecules must be monitored during degradation experiments. Our laboratory has contributed to analysis of molecule sizes by developing a permeation coefficient model (Logan & Jiang 1990) for analyzing particle size distributions.
Research into this area has gone on for many years in the Logan laboratory, primarily in the following areas: 1- characterizing molecular size distributions using ultrafiltration (UF) separation techniques; 2- microbial degradation of macromolecules; 3- removal of macromolecules in water and wastewater treatment processes. This work is described in a series of papers– see the publications link in the menu to the left. Additional detail on the permeation coefficient model can be found in Chapter 3 of my book Environmental Transport Processes, and the powerpoint presentation listed above (see UF-method).
Pictures
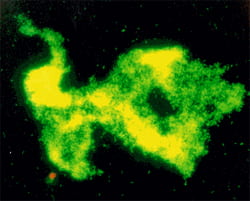
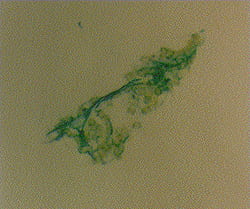
Bacterial aggregates formed by coagulation are not spheres, but rather fractals. This aggregate was stained with acridine orange and it fluoresces green.
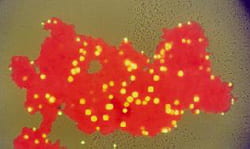
This is a photograph of a floc formed from red latex microspheres that has captured yellow-green microspheres during coagulation. The microspheres are latex particles. By counting the number of yellow-green beads in the red floc, we can determine collision efficiencies of fractal particles with other particles. This photograph was modified and used for the cover of the first addition of my textbook, Environmental Transport Processes (published by Wiley)
Selected Publications on Particles in the Environment
[For a complete list of publications, go to the publications page]
Serra, T. and B.E. Logan. 1999. Collision frequencies of fractal bacterial aggregates with small particles in a sheared fluid. Environ. Sci. Technol. 33(13):2247-2251.
Grossart, H.-P., M. Simon, and B.E. Logan. 1998. Formation of macroscopic organic aggregates (lake snow) in a large lake: The significance of transparent exopolymer particles, plankton and zooplankton. Limnol. Oceanogr. 42(8):1651-1672.
Li, X. U. Passow, and B.E. Logan. 1998. Fractal dimensions of small (15 to 200 µm) particles in eastern Pacific coastal waters. Deep-Sea Res. I, 45(1):115-131.
Jackson, G.A., R. Maffione, D.K. Costello, A.L. Alldredge, B.E. Logan, and H.G. Dam. Particle size spectra between 1 µm and 1 cm at Monterey Bay determined using multiple instruments. Deep-Sea Res. I, 44(11):1739-1767.
Li, X. and B.E. Logan. 1997. Collision Frequencies of Fractal Aggregates with Small Particles by Differential Sedimentation. Environ. Sci. Technol., 31(4):1229-1236.
Li, X. and B.E. Logan.. 1997. Collision Frequencies between Fractal Aggregates and Small Particles in a Turbulently Sheared Fluid. Environ. Sci. Technol., 31(4):1237-1242.
Johnson, C.P., X. Li and B.E. Logan. 1996. Settling velocities of fractal aggregates. Environ. Sci. Technol., 30(6):1911-1919.
Jiang, Q. and B.E. Logan. 1996. Fractal dimensions of aggregates produced in laminar and turbulent shear devices. J. AWWA. 88(2):100-113.
Logan, B.E. and J.R. Kilps. 1995. Fractal dimensions of aggregates formed in different fluid mechanical environments. Water Res. 29(2):443-453.
Logan, B.E., H.-P. Grossart, and M. Simon. 1994. Direct observation of phytoplankton, TEP, and aggregates on polycarbonate filters using brightfield microscopy. J Plankton Res., 16(12):1811-1815.
Logan, B.E. 1995. Comment on “Investigation of a sequential filtration technique for particle fractionation.” by Droppo et al, Environ. Sci. Technol., 29(8):2166-2167.
Jackson, G.A., B.E. Logan, A.L. Alldredge, and H. Dam. 1995. Combining particle size spectra from a mesocosm experiment measured using photographic and aperture impedance (Coulter and Elzone) techniques. Deep-Sea Res. II, 42(1):139-157.
Logan, B.E., U. Passow, A.L. Alldredge, H.-P. Grossart, and M. Simon. 1995. Rapid formation and sedimentation of large aggregates is predictable from coagulation rates (Half-Lives) of transparent exopolymer particles (TEP). Deep-Sea Res. II, 42(1):203-214.
Li, X. and B.E. Logan. 1995. Size distributions and fractal properties of particles during a simulated phytoplankton bloom in a mesocosm. Deep-Sea Res. II, 42(1):125-138.
Logan, B.E., H.-P. Grossart, and M. Simon. 1994. Direct observation of phytoplankton, TEP, and aggregates on polycarbonate filters using brightfield microscopy. J Plankton Res., 16(12):1811-1815.
Kilps, J.R., B.E. Logan, and A.L. Alldredge. 1994. Fractal dimensions of marine snow determined from image analysis of in situ photographs. Deep-Sea Res. 41(8):1159-1169.
Logan, B.E., U. Passow and A.L. Alldredge. 1994. Variable retention of diatoms on screens during size separations. Limnol. Oceanogr. 39(2):390-395.
Passow, U., A.L. Alldredge, and B.E. Logan. 1994. The role of particulate carbohydrate exudates in the flocculation of diatom blooms. Deep-Sea Res. 41(2):335-357.
Alldredge, A.L., Passow, U, and B.E. Logan. 1993. The abundance and significance of a class of large, transparent organic particles in the ocean. Deep-Sea Res. 40(6):1131-1140.
Logan, B.E. 1993. Theoretical analysis of size distributions determined using screens and filters. Limnol. Oceanogr. 38(2):372-381.
Jiang, Q. and B.E. Logan.. 1991. Fractal dimensions of aggregates determined from steady-state size distributions. Environ. Sci. Technol., 25(12), 2031-2038.
Logan, B.E. and D.B. Wilkinson. 1991. Fractal dimensions and porosities of Zoogloea ramigera and Saccharomyces cerevisae aggregates. Biotechnol. Bioengin., 38(4):389-396.
Logan, B.E, and Q. Jiang. 1990. A Model for determining molecular size distributions of DOM. J. Envir. Engin. Div., ASCE, 116(6):1046-1062.
Logan, B.E. and J.W. Dettmer. 1990. Increased mass transfer to microorganisms with fluid motion. Biotechnol. Bioengin., 35(11):1135 -1144.
Logan, B.E. and D.B. Wilkinson. 1990. Fractal geometry of marine snow and other biological aggregates. Limnol. Oceanogr., 35(1):130-136.
Logan, B.E. and A.L. Alldredge. 1989. The increased potential for nutrient uptake by flocculating diatoms. Mar. Biol. 101(4):443-450.
Logan, B.E. and J.R. Hunt. 1988. Bioflocculation as a microbial response to substrate limitations. Biotechnol. Bioeng., 31:91-101.
Logan, B.E. and J.R. Hunt. 1987. Advantages of microbial growth in permeable aggregates in marine systems. Limnol. Oceanogr., 32(5):1034-1048.
Selected Publications on Molecular Size Spectra
For a complete list of publications, go to the publications page]
Logan, B.E. and G. Wagenseller. 2000. Molecular size distribution of organic matter in wastewater transformed by treatment in a full scale trickling filter. Water Environ. Res. 72(3):277-281.
Confer, D.R. and B.E. Logan. 1998. A conceptual model describing macromolecule degradation by suspended cultures and biofilms Water Sci. Technol. 37 (4-5):231-234.
Confer, D.R., and B.E. Logan. 1998. Location of protein and polysaccharide hydrolytic activity in suspended and biofilm wastewater cultures. Wat. Res., 32(1):31-38.
Confer, D.R. and B.E. Logan. 1997. Molecular weight distribution of hydrolysis products during biodegradation of model macromolecules in suspended and biofilm cultures I: Bovine serum albumin. Wat. Res., 31(9):2127-2136.
Confer, D.R. and B.E. Logan. 1997. Molecular Weight Distribution of Hydrolysis Products during Biodegradation of Model Macromolecules in Suspended and Biofilm Cultures II: Dextran and Dextrin. Wat. Res., 31(9):2127-2145.
Confer, D.R., B.E. Logan, B.S. Aiken, and D.L. Kirchman. 1995. Measurement of dissolved free and combined amino acids in unconcentrated wastewaters using HPLC. Wat. Environ. Res. 67(1)118-125.
Haldane, G.M., and B.E. Logan. 1994. Molecular size distributions of a macromolecular polysaccharide (dextran) during its biodegradation in batch and continuous cultures. Wat. Res. 28(9):1873-1878.
Logan, B.E. and R.C. Fleury. 1993. Multiphasic kinetics can be an artifact of the assumption of saturable kinetics for microorganisms. Mar. Ecol. Prog. Ser. 102:115-124.
Logan, B.E. and D.K. Kirchman. 1991. Increased uptake of dissolved organics by marine bacteria as a function of fluid motion. Mar. Biol., 111(1):175-181.
Confer, D.R. and B.E. Logan. 1991. Increased bacterial uptake of macromolecular substrates with fluid shear. Appl. Environ. Microbiol., 57(11)3093-3100.
Logan, B.E. and Q. Jiang. 1990. A Model for determining molecular size distributions of DOM. J. Envir. Engin. Div., ASCE, 116(6):1046-1062.
Logan, B.E. and J.W. Dettmer. 1990. Increased mass transfer to microorganisms with fluid motion. Biotechnol. Bioengin., 35(11):1135 -1144.
Logan, B.E. and A.L. Alldredge. 1989. The increased potential for nutrient uptake by flocculating diatoms. Mar. Biol. 101(4):443-450.
Logan, B.E. and J.R. Hunt. 1988. Bioflocculation as a microbial response to substrate limitations. Biotechnol. Bioeng., 31:91-101.
Logan, B.E. and J.R. Hunt. 1987. Advantages of microbial growth in permeable aggregates in marine systems. Limnol. Oceanogr., 32(5):1034-1048.